A Novel, Simple, Accurate Method For Experimental Determination of Thermal Diffusivity: Measure 9 Types of Foods
Abstract:
Thermal diffusivity is an important material property, expressed as α = k/ρc, where α is the thermal diffusivity in m2/s, ρ is the density (kg/m3) and c is the specific heat (J/(kg ∙ K)). It is the core parameter that plays a key role in the heat transfer process described by the heat transfer equation. The equation describes one of the most important physical phenomena governing the world – heat transfer and exchange.Being able to accurately measure the thermal diffusivity of a material is important since the thermal diffusivity is an important material parameter that is essential to understand materials’ thermal properties and further in materials’ various applications. Thus, a consistent, simple, and low-cost measurement method is thus highly desirable.
Thermal diffusivity is often measured with the flash method, which is a method for the determination of the thermal diffusivity of different materials. It involves heating a strip or cylindrical sample with short energy, such as laser, pulses at one end, and analyzing the temperature change, i.e., reduction in amplitude and phase shift of the pulse, a short distance away. For instance, the sample is subjected to a high-intensity short duration radiant energy pulse. The power source can be a laser or a flash lamp. The energy will then be absorbed by the specimen and emitted again on the top of the sample. This radiation results in a temperature rise on the surface of the sample. This temperature rise is recorded from an infrared (IR) detector. The detector signal shows the duration of the measurement and the normalized temperature rise on the surface of the sample, where the light pulse occurs. For the calculation of the thermal diffusivity, it is necessary to determine the baseline and the maximum temperature rise. This is done by a suited fitting model. Additionally, the model determines the time at which half of the maximum temperature rise was reached. Such a method requires expensive instruments and a more complex process. Other alternative methods also involve expensive and complex equipment and process.
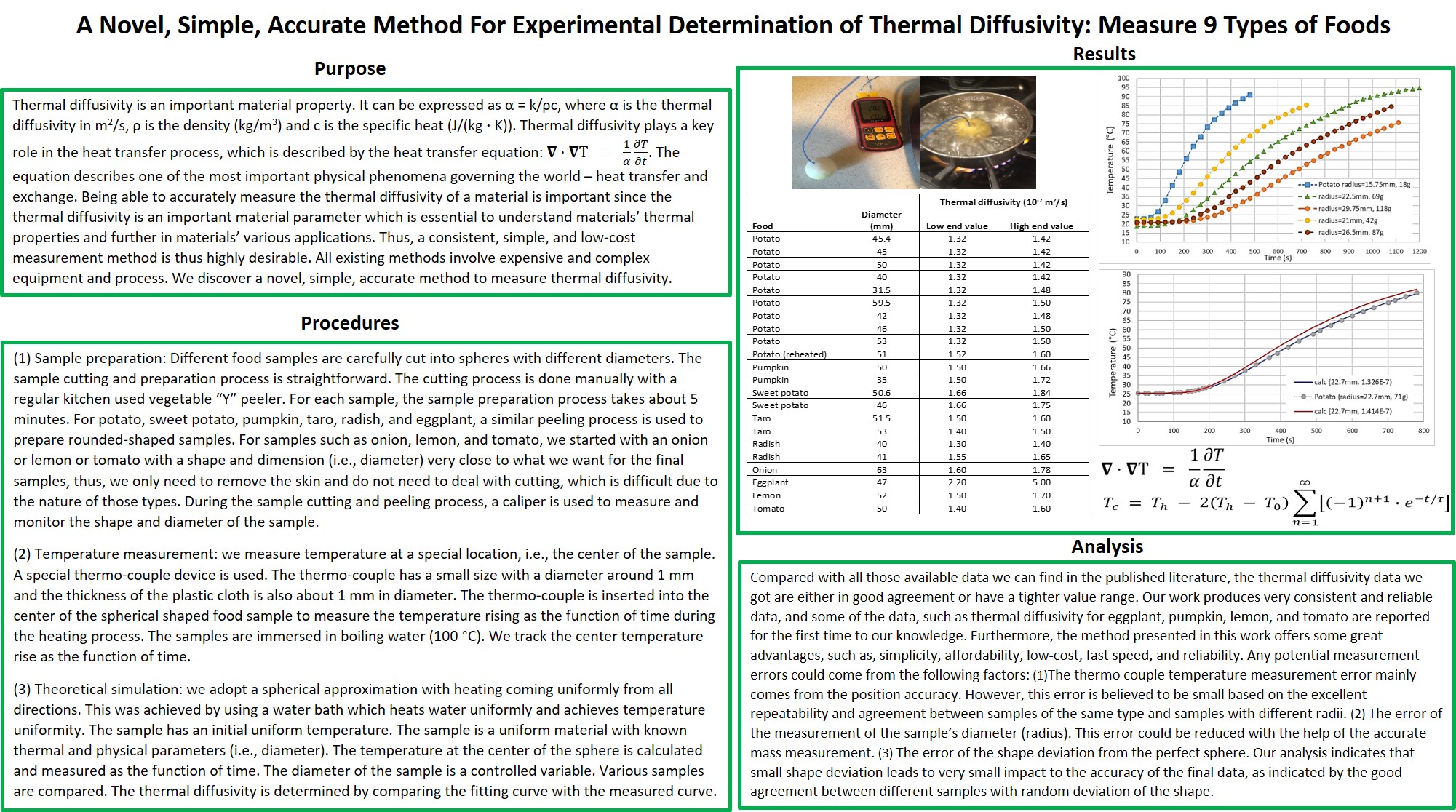
In this work, we developed a novel, simple, low-cost, and straightforward method to measure the thermal diffusivity of various foods. 9 different types of foods are studied and their thermal diffusivities are experimentally determined. The foods which are studied include potato, sweet potato, pumpkin, taro, radish, eggplant, lemon, tomato, and onion. We pre-cut the foods into a spherical shape and then insert a small and thin thermocouple sensor to the center of the spherically-shaped food, and immerse the food samples in the boiling water. The center temperature is recorded throughout the heating process. We then compare the heating curve as the function of time with the simulation results, where thermal diffusivity is used as the fitting parameter. This method allows us to intentionally vary the diameter of the spheres, i.e., adding a controlled parameter in order to validify the results. We are able to determine all the thermal diffusivity data with good consistency between the measurement data and the simulation results. This method can be generalized to determine thermal diffusivity for other types of materials.
We have repeated the measurements for potatoes with multiple samples. The results we obtained are very consistent, which indicates the consistency of this measurement method. The obtained result for potato is between 1.32x10-7 m2/s and 1.50x10-7 m2/s with a tight range, and the result is in excellent agreement with some of the reported values, and in good agreement with all the other reported values.
Compared with all those available data we can find in the published literature, the thermal diffusivity data we got are either in the good agreement or have a tighter value range. Compared to all the published thermal diffusivity data we can find, our work produces very consistent and reliable data, and some of the data, such as thermal diffusivity for eggplant, pumpkin (not pumpkin seed), lemon (not lemon juice), and tomato (not tomato pulp or paste) are reported for the first time to our knowledge. Furthermore, the method presented in this work offers some great advantages, such as simplicity, affordability, low-cost, fast speed, and reliability. Any potential measurement errors could come from the following factors. (1) The thermocouple temperature measurement error mainly comes from the position accuracy. However, this error is believed to be small based on the excellent repeatability and agreement between samples of the same type and samples with different radii. (2)The error of the measurement of the sample’s diameter (radius). This error could be reduced with the help of accurate mass measurement. (3) The error of the shape deviation from the perfect sphere. Our analysis indicates that a small shape deviation leads to a very small impact on the accuracy of the final data, as indicated by the good agreement between different samples with a random deviation of the shape.
Bibliography/Citations:
- B.R. Becker, B.A. Fricke, Thermal Properties of Foods, in Encyclopedia of Food Sciences and Nutrition (Second Edition), 2003.
- N. N. Mohsenin, Thermal properties of Foods and Agricultural Materials. Gordon and Breach Science Publishers, New York, (1980).
- V. E. Sweat, Thermal Properties of Foods. In: Engineering Properties of Foods, Ed.: M. A. Rao, S. S. H. Rizvi, M. Dekker, NY-Basel, pp. 99-138, (1995).
- W.J. Parker; R.J. Jenkins; C.P. Butler; G.L. Abbott (1961). "Method of Determining Thermal Diffusivity, Heat Capacity and Thermal Conductivity". Journal of Applied Physics 32, 1679 (1961); https://doi.org/10.1063/1.1728417
- Dickerson, R.W. 1965. An Apparatus for the Measurement of the Thermal Diffusivity of Foods. Food Technol., 19: 198–204.
- K.Porsdal Poulsen, Thermal diffusivity of foods measured by simple equipment, Journal of Food Engineering, Volume 1, Issue 2, 1982, Pages 115-122
- J. Blumm; J. Opfermann (2002). "Improvement of the mathematical modeling of flash measurements". High Temperatures – High Pressures. 34 (5): 515. doi:10.1068/htjr061
- Thermitus, M.-A. (October 2010). "New Beam Size Correction for Thermal Diffusivity Measurement with the Flash Method". In Gaal, Daniela S.; Gaal, Peter S. (eds.). Thermal Conductivity 30/Thermal Expansion 18. 30th International Thermal Conductivity Conference/18th International Thermal Expansion Symposium. Lancaster, PA: DEStech Publications. p. 217. ISBN 978-1-60595-015-0. Retrieved 1 December 2011.
- Edward A. Olszewskia, From baking a cake to solving the diffusion equation, American Journal of Physics 74, 502 (2006); https://doi.org/10.1119/1.2186330,.
- H. Nelson, S. Deyo, S. Granzier-Nakajima, P. Puente, K. Tully, J. Webb, A mathematical model for meat cooking, Eur. Phys. J. Plus (2020) 135:322, https://doi.org/10.1140/epjp/s13360-020-00311-0.
- H. C. Chang, J. A. Carpenter, R. T. Toledo, Modeling heat transfer during oven roasting of unstuffed turkeys, Journal of Food Science, Vol. 63, Issue 2, June 2008. https://doi.org/10.1111/j.1365-2621.1998.tb15721.x
- Jan Taler, Paweł Ocłoń, Transient Heat Conduction in Sphere, January 2014, DOI: 10.1007/978-94-007-2739-7_400, In book: Encyclopedia of Thermal Stresses (pp.6186-6198).
- M.E. Shmalko, R. O. Morawicki, and L. A. Ramallo, Simultaneous determination of specific heat and thermal conductivity using the finite-difference method, Journal of Food Eng. 31, 531-540, (1996).
- Srivastava A.K, Shukla S.K, Singh U.K, “Modeling and Evaluation of Thermal Diffusivity and Activation Energy of Potato slices in Forced Convection Multi Tray Solar Dryer”, American Journal of Food Science and Technology, 2015, Vol. 3, No. 2, 27-32, Available online at http://pubs.sciepub.com/ajfst/3/2/1
- T.R.A.Magee and T.Bransburg, “Measurement of thermal diffusivity of potato, malt bread and wheat flour”, Journal of Food Engineering, Volume 25, Issue 2, 1995, Pages 223-232.
- CHAPTER 9, THERMAL PROPERTIES OF FOODS, 2006 ASHRAE Handbook—Refrigeration (SI)
- Mathews, F.W., Jr. and C.W. Hall. 1968. Method of finite differences used to relate changes in thermal and physical properties of potatoes. ASAE Transactions 11(4):558.
- Minh, T.V., J.S. Perry, and A.H. Bennett. 1969. Forced-air precooling of white potatoes in bulk. ASHRAE Transactions 75(2):148-150.
- Riedel, L. 1969. Measurements of thermal diffusivity on foodstuffs rich in water. Kaltetechnik 21(11):315-316.
- Sweat, V.E. 1985. Thermal properties of low- and intermediate-moisture food. ASHRAE Transactions 91(2):369-389.
- Adefemi Farinu & Oon-Doo Baik (2007) Thermal Properties of Sweet Potato with its Moisture Content and Temperature, International Journal of Food Properties, 10:4, 703-719, DOI: 10.1080/10942910601137482
- Kotecha, P.M.; Kadam, S.S. Sweet potato. In Handbook of Vegetable Science and Technology: Production, Composition, Storage and Processing; D.K. Salunkhe; S. S. Kadam.; Eds. Marcel Dekker; New York, 1998, 71–98.
- Choi, Y.; Okos, M.R. Effects of Temperature and Composition on the Thermal Properties of Foods. In Food Engineering and Process Applications, Vol. 1; Lemaguer, M.; Jelen, P.; Eds.; Elsevier, London, 1985; 93.
- Krishna Kumar, P., Bhunia, K., Tang, J., Rasco, B. A., Takhar, P. S., & Sablani, S. S. (2018). Thermal transition and thermo-physical properties of potato (Solanum tuberosum L.) var. Russet brown. Journal of Food Measurement and Characterization, 12(3), 1572-1580. https://doi.org/10.1007/s11694-018-9772-x
- Yifei “Jenny” Jin, Lisa R. Wang, and Jian Jim Wang, Physics in turkey cooking: Revisit the Panofsky formula, AIP Advances 11, 115316 (2021); https://doi.org/10.1063/5.0067811
- T.R.A. Magee and T. Bransburg, “Measurement of thermal diffusivity of potato, malt bread and wheat flour”, Journal of Food Engineering, Volume 25, Issue 2, 1995, Pages 223-232. https://doi.org/10.1016/0260-8774(94)00025-5.
- M. A. Rao, J. Barnard, J. F. Kenny, “Thermal Conductivity and Thermal Diffusivity of Process Variety Squash and White Potatoes,” Transactions of the ASAE. 18 (6): 1188-1192. (doi: 10.13031/2013.36767)
- Engineering ToolBox, (2020). Foodstuff - Thermal Diffusivity. https://www.engineeringtoolbox.com/foodstuff-thermal-diffusivity-d_2176.html.
- A. Farinu and O. Baik, “Thermal Properties of Sweet Potato with its Moisture Content and Temperature,” International Journal of Food Properties, 10:4, 703-719, (2007) DOI: 10.1080/10942910601137482.
- M.S. Obot, C. Li, T. Fang, J. Chen, “Measurement of thermal properties of white radish using easily constructed probes,” PLoS ONE 12(3): e0171016. (2017) doi:10.1371/journal.pone.0171016.
- H. Kocabiyik, B. Kayisoglu and D. Tezer, “Effect of Moisture Content on Thermal Properties of Pumpkin Seed,” International Journal of Food Properties, 12:2, 277-285, (2009) DOI: 10.1080/10942910701673519.
- A.E. Drusas and G.D. Saravacos, “Thermal conductivity of tomato paste,” Journal of Food Engineering, Volume 4, Issue 3, 1985, Pages 157-168.
- L. Abhayawick, J. Laguerre, V. Tauzin, A. Duquenoy, “Physical properties of three onion varieties as affected by the moisture content,” Journal of Food Engineering. 55. 253-262. (2002) 10.1016/S0260-8774(02)00099-7.
- L. A. Minim, V. R. N. Telis, V. P. R. Minim, L. A. P. Alcantara, and J. Telis-Romero, “Thermophysical Properties of Lemon Juice as Affected by Temperature and Water Content,” J. Chem. Eng. Data 2009, 54, 8, 2269–2272, 2009. https://doi.org/10.1021/je900155c
Additional Project Information
Research Plan:
The existing methods to experimentally determine the thermal diffusivity of material usually involve expensive and complex equipment and process. The purpose of this research is to explore a simple experimental method to determine thermal diffusivity. I hope the new method can be reliable, consistent, simple, low-cost, fast, and accurate. I also want to apply this new method to measure the thermal diffusivity of various foods. Of course, this method can be generalized to determine thermal diffusivity for other types of materials.
The goal of this project is to develop a new method to experimentally measure the thermal diffusivity of various food materials, such as potato, radish, taro, etc. The new method we hope to develop from this research is expected to be simple, fast, accurate, and low-cost.
The research contains the following main steps and processes:
(1) Literature search and research and study to understand the pre-existing methods to determine the thermal diffusivity. We will conduct a detailed and extensive literature search to fully understand the existing methods for measuring thermal diffusivity.
(2) Understand thermal transfer physics, all physical parameters, and its physical description and mathematics. We need to clearly understand the thermal transfer process and its physics description and mathematical equations. It includes all the physical parameters and their meanings and implications.
(3) Find a simple and accurate method to measure the temperature change within the sample, particularly, all the food samples. Whatever method we develop will involve accurate temperature measurement. We need to find a simple, low-cost, and accurate method to measure temperature and temperature changes.
(4) Understand, acquire, purchase, characterize and familiarize with all the tools, temperature measurement equipment, samples, and other experimental needs. All the tools, equipment, and materials are purchased and acquired in this step.
(5) Experimentally measure the heating curves for various samples, with the diameter as the main variable. We plan to conduct the experiment in a home kitchen and measure the heating curve (i.e., the temperature curve) for all the samples. The samples include various food materials and different physical dimensions, e.g., the diameter.
(6) Mathematically solve the heat transfer equation for a spherical configuration. To theoretically understand the experiment, we will need to solve the heat transfer equation based on the real configuration.
(7) Fitting the measurement curves with the theoretical simulations with thermal diffusivity as the fitting parameter.
(8) Analyze the obtained thermal diffusivity data and compare them with any published ones on literature that are available.
(9) Analyze any possible errors and deviations.
(10) Write research reports and papers.
Questions and Answers
1. What was the major objective of your project and what was your plan to achieve it?
a. Was that goal the result of any specific situation, experience, or problem you encountered?
b. Were you trying to solve a problem, answer a question, or test a hypothesis?
I love cooking all sorts of dishes. As a result of my interest in cooking and science, I have been often intrigued by the sciences in culinary arts. The problems I have tried to answer include: why does it only take a few minutes to prepare a dish in a Chinese restaurant? however, it takes 30-60 mins to prepare a steak in an American restaurant? I have been studying cooking-related science, particularly, physics, for a while. After I understand more about the thermal transfer process and the physics behind it, I wanted to explore more deeply. In my previous research, I have studied the physics behind the turkey baking process, for instance.
Thermal diffusivity is an important material property, the core parameter which plays a key role in the heat transfer process. Being able to quickly and accurately measure the thermal diffusivity of a material is important since thermal diffusivity is an important material parameter that is essential to understand materials’ thermal properties and further in materials’ various applications. Thus, a consistent, simple, and low-cost measurement method is thus highly desirable. Thermal diffusivity is often measured with the flash method, which is a method for the determination of the thermal diffusivity of different materials. It involves heating a strip or cylindrical sample with short energy, such as laser, pulses at one end and analyzing the temperature change, i.e., reduction in amplitude and phase shift of the pulse, a short distance away. This radiation results in a temperature rise on the surface of the sample. This temperature rise is recorded from an infrared (IR) detector. Such a method requires expensive instruments and a more complex process. Other alternative methods also involve expensive and complex equipment and process. My purpose for this research is to explore a simple experimental method to determine thermal diffusivity. I hope the new method can be reliable, consistent, simple, low-cost, fast, and accurate. I also want to apply this new method to measure the thermal diffusivity of various foods. Of course, this method can be generalized to determine thermal diffusivity for other types of materials.
2. What were the major tasks you had to perform in order to complete your project?
a. For teams, describe what each member worked on.
(1) Literature search and research and study to understand the pre-existing methods to determine the thermal diffusivity.
(2) Understand thermal transfer physics, all physical parameters, and its physical description and mathematics.
(3) Find a simple and accurate method to measure the temperature change within the sample, particularly, all the food samples.
(4) Understand, acquire, purchase, characterize and familiarize with all the tools, temperature measurement equipment, samples, and other experimental needs.
(5) Experimentally measure the heating curves for various samples, with the diameter as the main variable.
(6) Mathematically solve the heat transfer equation for a spherical configuration.
(7) Fitting the measurement curves with the theoretical simulations with thermal diffusivity as the fitting parameter.
(8) Analyze the obtained thermal diffusivity data and compare them with any published ones on literature that are available.
(9) Analyze any possible errors and deviations.
(10) Write research reports and papers.
3. What is new or novel about your project?
a. Is there some aspect of your project's objective, or how you achieved it that you haven't done before?
b. Is your project's objective, or the way you implemented it, different from anything you have seen?
c. If you believe your work to be unique in some way, what research have you done to confirm that it is?
We discovered a novel, simple, low-cost, and straightforward method to measure the thermal diffusivity of various foods. 9 different types of foods are studied and their thermal diffusivities are experimentally determined. The foods which are studied include potato, sweet potato, pumpkin, taro, radish, eggplant, lemon, tomato, and onion. We pre-cut the foods into a spherical shape and then insert a small and thin thermocouple sensor to the center of the spherically-shaped food, and immerse the food samples in the boiling water. The center temperature is recorded throughout the heating process. We then compare the heating curve as the function of time with the simulation results, where thermal diffusivity is used as the fitting parameter. This method allows us to intentionally vary the diameter of the spheres, i.e., adding a controlled parameter in order to validify the results. We are able to determine all the thermal diffusivity data with good consistency between the measurement data and the simulation results. This method can be generalized to determine thermal diffusivity for other types of materials.
Our method is new, unique, and novel. Based on our extensive literature research, we haven't found any similar method in any literature or online searches.
4. What was the most challenging part of completing your project?
a. What problems did you encounter, and how did you overcome them?
b. What did you learn from overcoming these problems?
The most challenging part of this project is the theoretical analysis and mathematical derivation of the formula used in our simulation. It requires some extensive knowledge in calculus and multi-variable calculus. I had to seek helps from my mentor and many other people. What I learned through solving this problem is that studying science needs more discussions. Talking to other people really help me understand more and learn more.
5. If you were going to do this project again, are there any things you would do differently the next time?
Not really. But we do want to expand this method to measure other materials such as plastics, meat, etc.
6. Did working on this project give you any ideas for other projects?
During the work of this project, we came up with a new idea for another project, that is, to experimentally measure the diffusivity of salt, for instance. This project also allowed me to deeply understand the intelligence in different types of cooking, another very fascinating project.
7. How did COVID-19 affect the completion of your project?
The COVID-19 didn't affect the completion of this project since I performed this project completely at home including all the experiments.